Pyramid Calculator

The figure below shows these two kinds of pyramids.

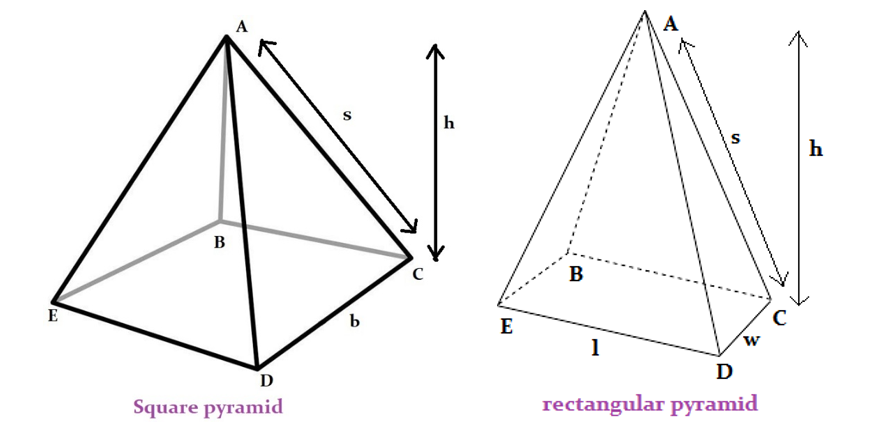
In both these figures, the vertex is A. In the square pyramid, the base is a square of side ‘b’. In the rectangular pyramid, the base is a rectangle of length ‘l’ and width ‘w’. The height of the pyramid is the distance between the base and the vertex, denoted by ‘h’. The slant height of the pyramid is the distance between the vertex and one edge of the base along a line segment in the triangle connecting these two. In the figures above, the slant height from the vertex A to one of the sides CD has been marked as ‘s’.

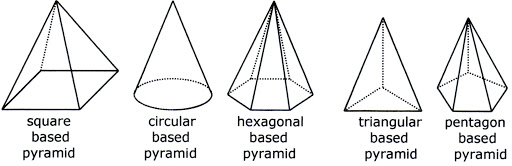
Apart from the square and rectangular pyramids, there may be other types of pyramids named after the polygon at the base. This polygon may be a square, rectangle, triangle, pentagon, hexagon, circle and more. This has been illustrated below. The pyramid having a circular base is essentially a cone.

Given below are some properties of a pyramid.

Volume – This is the total space occupied by the pyramid.

Lateral surface area – The lateral surface area of a pyramid is the area excluding the surface area of the base. It is the area of the slanted portion of the pyramid.

Surface area – Surface area is the total area covered by the pyramid. It is equal to the sum of the lateral surface area and the area covered by the polygon at the base.

Given below are the formulas of a pyramid.

The general formula for the volume and surface area of a pyramid is as follows:

Surface area = base area + 1/2 (number of sides in the base × slant height×length of the base)

Volume = 1/3 x base area × height

For a square pyramid:

Here, b indicates the length of the base, h is the height of the pyramid, and s is the slant height.

For a rectangluar pyramid:

Here, l indicates the length of the base, w is the width, h is the height of the pyramid, and s is the slant height.

Given below are the main characteristics of a pyramid.

A pyramid is a polyhedron having a polygon at its base, the corners of which are connected to the vertex.

The vertex and the edge of the base form a triangle on each side of the pyramid.

Pyramids are named after the polygon at their base.

Square pyramids have a square at their base, whereas rectangular pyramids have a rectangle at their base.

The volume of the pyramid is the total region occupied by it in three-dimensional space.

The lateral surface area of the pyramid is the area of the slanted sides, and excluding the area of the base.

The total surface area is the sum of the lateral surface area and the area of the base.

If the base of a pyramid is a regular polygon (like square, rectangle, pentagon, hexagon, triangle, etc.), it is called a regular pyramid. Otherwise, it is considered an irregular pyramid.

A pyramid is called a right pyramid if the vertex lies exactly over the middle of the base.

When the vertex of the pyramid does not lie directly above the middle of the base, it is called an oblique pyramid.

These two kinds of pyramids have been shown below.

Pyramids are not very common in everyday life. However, in Egyptian culture, they are symbolically significant, so many designers and artists incorporate the pyramidal shape in their artwork, sculpture or architecture. The most notable example of pyramids are the pyramids of Giza in Egypt, the Great American Pyramid in Memphis, USA and the glass Louvre pyramid in Paris, France. Some other commonly observed pyramids are cheese graters and the slanted roofs of houses.

We now consider an example making use of the pyramid formulas.

---

---