Mixed Fraction Simplifier - simplify the mixed fraction number
Mixed Fraction Simplifier
Mixed Fraction Number
Result Summary:
| Type | Result |
| Given Fraction number | 0 |
| Simplified Fraction number | 0 |
What is a mixed Fraction?
A fraction is a number of the form a/b, where b ≠ 0. It represents some part of a whole. Fractions are of three types: Proper fraction, improper fraction and mixed fraction.
In a proper fraction, the numerator is lesser than the denominator, whereas it is the opposite for an improper fraction. A mixed fraction (also called a mixed number) is a fraction which has a whole part and a fractional part. For example, 2 ¼ is a mixed fraction, where 2 is the whole number and ¼ is the fractional part.
For performing any calculations, the mixed fractions need to be converted to improper fractions first. For example, 2 ¼ in mixed fraction form is 9/4. Consider an example of a mixed fraction below.
Consider a pizza cut into 4 slices. Suppose one whole pizza and one half of another pizza have been given as shown here. The one whole pizza represents the whole number, which is 1 in this case, and ½ is the fractional part. Combining these two together gives the mixed fraction 1 ½, which indicates one whole pizza and ½ part of another pizza.
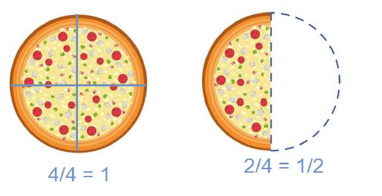
Now, if there were two whole pizzas present along with the half pizza, the whole number would be 2, and the fractional number would be ½. In mixed fraction form, this is 2 ½.
Mixed fractions, like all other types of fractions, obey all the usual properties of fractions such as commutative property under addition and multiplication, and the distributive property. The inverse of a mixed fraction is the reciprocal of the corresponding improper fraction, and the identity element is 0 (under addition) and 1 (under multiplication).
Converting a mixed fraction to an improper fraction
Consider the mixed fraction 1 2/5. We try to convert this mixed fraction into an improper fraction.
1) First, we multiply the whole number by the denominator. This gives us 5.
2) Now we add up this 5 with the numerator, to get 7. 7 is the numerator of the improper fraction.
3) The denominator stays the same. The corresponding improper fraction is 7/5.
Converting an improper fraction to a mixed fraction
Consider the fraction 12/8. We try to convert this improper fraction into a mixed fraction.
1) The first thing we might observe is that the fraction is not in its simplest form. If that is the case, then the fraction needs to be reduced to its simplest form before it is converted to a mixed fraction.
2) For the fraction 12/8, the GCD of 12 and 8 is 4. On dividing both the numerator and denominator by 4, we get 3/2. This is the simplest form of the improper fraction.
3) The above step can be skipped if the improper fraction is already in its simplest form.
4) We now convert the improper fraction to a mixed fraction. Divide the numerator by the denominator.
5) Note down the quotient after division. This is the whole number of the mixed fraction. When 3 is divided by 2, the quotient is 1.
6) The remainder obtained after division becomes the numerator of the mixed fraction. The remainder obtained after dividing 3 by 2 is 1.
7) The denominator stays the same, which is 2.
8) Hence, the resulting mixed fraction is 1 1/2.
Simplifying mixed fractions
Most of the mixed fractions we encounter are expressed in their simplest form. This means that the fractional part of the mixed fraction is in its simplest form. If the fractional part is not in its simplest form, it can be changed into one using the following steps.
Consider the mixed fraction 5 6/12.
1) The first thing we might observe is that the fractional part is not in its simplest form. We need to convert it to the simplest form.
2) For this, consider the fractional part 6/12. The GCD of 6 and 12 is 6.
3) Divide the numerator and denominator by 6. This reduces the fraction to ½.
4) Replace the 6/12 in the mixed fraction with ½.
5) Hence, the simplified mixed fraction is 5 1/2.
Areas of application
Mixed fractions and improper fractions are frequently used in mathematical computations. Consider the following problem making use of mixed fractions.
Question: Jonah walked 2 2/3 miles on Saturday and 3 2/5 miles on Sunday. What is the total distance he walked over the weekend?
Answer: To find the total distance Jonah walked, we first need to convert the mixed fractions into improper fractions.
2 2/3 miles and 3 2/5 miles can be written as 8/3 miles and 17/5 miles.
To add up these fractions, we first find the LCM of the denominators. The LCM of 3 and 5 is 15. $$ {8\over3}+{17\over5} $$
Now we multiply the fractions with the numbers so that the denominator multiplied by these numbers gives the LCM. Hence, the first fraction is multiplied by 5 and the second one by 3.
$$ {{8*5}\over{3*5}}+{{17*3}\over{5*3}} = {40\over15}+{51\over15} = {{40+51}\over15} = {91\over15} = 6{1\over15} $$
The total distance covered by Jonah over the weekend is 91/15 miles. As a mixed fraction, this distance is 6 1/15 miles.

